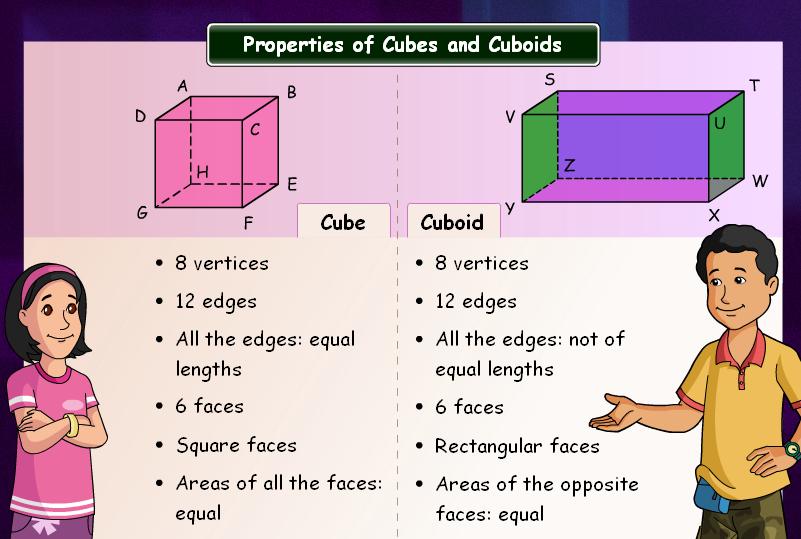
1. In a cube or a cuboid there are six faces in each.
2. In a cube length, breadth and height are same while in cuboid these are different.
3. In a cube the number of unit cubes = $(side)^{3}$ and in cuboid the number of unit cube = $(l\times b\times h)$

Example on Cube and Cuboid

A cube of each side 4 cm, has been painted black, red and green on pairs of opposite faces. It is then cut into small cubes of each side 1 cm.

Then find

1. How many small cubes will be there ?
2. How many small cube will have three faces painted ?
3. How many small cubes will have only two faces painted ?
4. How many small cubes will have only one faces painted ?
5. How many small cubes will have no faces painted ?
6. How many small cubes will have only two faces painted black and green and all other faces unpainted ?
7. How many small cubes will have only two faces painted green and red.
8. How many small cubes will have only two faces painted black and red ?
9. How many small cubes will be only black painted ?
10. How many small cubes will be only red painted ?
11. How many small cubes will be only green painted ?
12. How many small cubes will have atleast one face painted ?
13. How many small cubes will have atleast two faces painted ?

The solution of each question given above, is given below with explanation. Here three faces are visible.

Steps :

(1) A cube of each side 4 cm is here.

(2) It is cut into small cubes of each side of 1 cm.

(3) Opposite faces are painted with black, red and green paints.

1. Total no. of small cubes-$(S)^{3}\: =\: (4)^{3}$ = 64.

2. No. of small cubes having three faces painted- From the figure it is clear that the small cube having three faces coloured are situated at the corners of the big cube because at these corners only three faces of the big cube meet. Therefore the required number of such cubes is always 8, because there are 8 corners.

3. No. of small cubes having only two faces painted- From the figure it is clear that to each edge of the big cube 4 small cubes are connected and two out of them are situated at the corners of the big cube which have all the three faces painted. Thus, to each edge two small cubes are left which have two faces painted. As the total no. of edges in a cube are 12, hence the no. of small cubes with two faces coloured
= $12\times 2$ = 24.

Or

No. of small cubes with two faces coloured
= $(x-2)\times \: No.\: of \: edges$
Where x = $\frac{Side\: of\: big\: cube}{Side\: of \: small\: \: cube}$

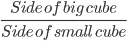
4. No. of small cubes having only one face painted- The cubes which are painted on one face only are the cubes at the centre of each face of the big cube. Since there are 6 faces in the big cube and each of the face of big cube there will be four such small cubes. Hence, in all there will be 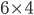$6\times 4$ i.e., 24 such small cubes.
or 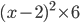$(x-2)^{2}\times 6$

5. No. of small cubes having no face painted- No. of such small cubes
= $(x-2)^{3}\: \: \left [ Hence,\: x\: =\: \frac{4}{1} \: =\: 4\right ]$
= $(4-2)^{3}\: =\: 8$

6. No. of small cubes having two faces painted black and green- There are 4 small cubes in layer II and 4 small cubes in layer III which have two faces painted green and black.
$\therefore$ Reqd. no. of such small cubes = 4 + 4 = 8.

7. No. of small cubes having two faces painted green and red.
Reqd. no. of such small cubes = 4 + 4 = 8.

8. No. of small cubes having two faces painted black and red = 4 + 4 = 8.

9. No. of small cubes having only black paint. There will be 8 small cubes which have only black paint. Four cubes will be from one side and 4 from the opposite side.

10. No. of small cubes having only red paint
= 4 + 4 = 8

11. No. of small cubes having only green paint
= 4 + 4 = 8

12. No. of small cubes having atleast one face painted
= No. of small cubes having 1 face painted + 2 faces painted + 3 faces painted
= 24 + 24 +8 = 56

13. No. of small cubes having atleast two faces painted
= No. of small cubes having two faces painted + 3 faces painted
= 24 + 8 = 32

Cube and Cuboid Questions from Previous Year Exams

Cube and Cuboid Video Lecture