Decimal Fraction Formulas
Decimal Fractions: Fractions in which denominators are powers of 10 are known as decimal fractions.
1/10 = 1 tenth = .1
1/100 = 1 hundredth = .01
9/100 = 99 hundredth = .99
7/1000 = 7 thousandths =.007
Conversion of a Decimal Into Vulgar Fraction : Put 1 in the denominator under the decimal point and annex with it as many zeros as is the number of digits after the decimal point.
Now, remove the decimal point and reduce the fraction to its lowest terms.Thus,
0.25 = 25/100 = 1/4
2.008 = 2008/1000 = 251/125.
Annexing zeros to the extreme right of a decimal fraction does not change its value.
0.8 = 0.80 = 0.800, etc.
If numerator and denominator of a fraction contain the same number of decimal places, then we remove the decimal sign.
1.84/2.99 = 184/299 = 8/13
0.365/0.584 = 365/584 = 5
Operations on Decimal Fractions :
1) Addition and Subtraction of Decimal Fractions : The given numbers are so placed under each other that the decimal points lie in one column. The numbers so arranged can now be added or subtracted in the usual way.
2) Multiplication of a Decimal Fraction By a Power of 10 : Shift the decimal point to the right by as many places as is the power of 10. Thus, 5.9632 x 100 = 596,32; 0.073 x 10000 = 0.0730 x 10000 = 730.
Multiplication of Decimal Fractions : Multiply the given numbers considering them without the decimal point. Now, in the product, the decimal point is marked off to obtain as many places of decimal as is the sum of the number of decimal places in the given numbers. Suppose we have to find the product (.2 x .02 x .002).
Now, 2x2x2 = 8. Sum of decimal places = (1 + 2 + 3) = 6. .2 x .02 x .002 = .000008.
Dividing a Decimal Fraction By a Counting Number : Divide the given number without considering the decimal point, by the given counting number. Now, in the quotient, put the decimal point to give as many places of decimal as there are in the dividend. Suppose we have to find the quotient (0.0204 + 17). Now, 204 ^ 17 = 12. Dividend contains 4 places of decimal. So, 0.0204 + 17 = 0.0012.
Dividing a Decimal Fraction By a Decimal Fraction: Multiply both the dividend and the divisor by a suitable power of 10 to make divisor a whole number. Now, proceed as above.
Thus,0.00066/0.11 = (0.00066*100)/(0.11*100) = (0.066/11) = 0.006V
Comparison of Fractions: Suppose some fractions are to be arranged in ascending or descending order of magnitude. Then, convert each one of the given fractions in the decimal form, and arrange them accordingly. Suppose, we have to arrange the fractions 3/5, 6/7 and 7/9 in descending order.
now,
3/5 = 0.6
6/7 = 0.857
7/9 = 0.777....
since 0.857 > 0.777...> 0.6
so 6/7>7/9>3/5
Recurring Decimal : If in a decimal fraction, a figure or a set of figures is repeated continuously, then such a number is called a recurring decimal. In a recurring decimal, if a single figure is repeated, then it is expressed by putting a dot on it. If a set of figures is repeated, it is expressed by putting a bar on the set, Thus
1/3 = 0.3333….= 0.3; 22 /7 = 3.142857142857.....= 3.142857
Pure Recurring Decimal: A decimal fraction in which all the figures after the decimal point are repeated, is called a pure recurring decimal.
Converting a Pure Recurring Decimal Into Vulgar Fraction : Write the repeated figures only once in the numerator and take as many nines in the denominator as is the number of repeating figures. thus ,
0.5 = 5/9; 0.53 = 53/59 ;0.067 = 67/999; etc...
Mixed Recurring Decimal: A decimal fraction in which some figures do not repeat and some of them are repeated, is called a mixed recurring decimal. e.g., 0.17333= 0.173.
Converting a Mixed Recurring Decimal Into Vulgar Fraction : In the numerator, take the difference between the number formed by all the digits after decimal point (taking repeated digits only once) and that formed by the digits which are not repeated, In the denominator, take the number formed by as many nines as there are repeating digits followed by as many zeros as is the number of non-repeating digits. Thus
0.16 = (16-1) / 90 = 15/19 = 1/6;
Decimal Fraction Solved Examples
Example 1: Convert (i) 0.75 and (ii) 2.008 into vulgar fractions.
Solutions:
i) 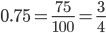
ii) 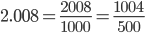
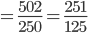
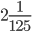
Rule - for Converting a decimal into a vulgar fractions: In the denominator put 1 under decimal point and annex with it as many zeros as the number of digits after the decimal point. Next , remove the decimal point and write whole number in numerator. Reduce it to lowest form.
Remark - Annexing zeros to the extreme right of a decimal fraction does not change its value.
Addition and subtraction of decimal fractions.
Example 2: Convert (i) 45.7 + 3.098 + 0.79 + 0.8 = ?
(ii) 9.053 – 3.69 = ?
Solution:
| i) 45.7 |
ii) 9.053 |
| + 3.098 | - 3.69 |
| +0.79 | 5.363 |
| +0.8 | |
| 50.388 |
Thus for addition the given numbers are so placed under each other that the decimal point lie in column. Now, the number can be added or subtracted as usual putting the decimal point under the decimal points.
Example 3: Evaluate i) 6.4209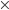 100 ii) 0.0379
100 ii) 0.0379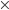 10
10
Solution:
1 ) 6.4209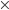 100 = 642.09
100 = 642.09
2) 0.0379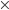 100 = 0.379
100 = 0.379
3) 0.009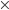 1000 = 9
1000 = 9
Thus when we multiply a decimal fraction by a power of 10, shift the decimal point to the right by as many places of decimal as is the power of 10.
Multiplication of two or more decimal fraction.
Example 4: Find the productions
1) 2.257 2.1
2.1
2) 2.79 1.31
1.31
3) 5 0.5
0.5 0.0005
0.0005
Solution:
(1) 2.257 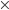 2.1 = 4.7397
2.1 = 4.7397
Sum the decimal place = (3 + 1) = 4
Now put the decimal after 4 that counting 4 digits from right thus 4.7397 is answer .
(2) 2.79  1.31 = 3.6549
1.31 = 3.6549
Multiplied 279 and 131, result is 36549. Total decimal places are 4, put decimal counting four digits from right.
(3) 5  0.5
0.5 0.05
0.05 0.0005 = 0.0000625
0.0005 = 0.0000625
Multiplied 5, 4 times, get 625, now number of decimal places are 7, so place 4 zeros on left of 625 and then mark decimal.
Decision of decimal fraction by a non zero whole number.
Example 5: Evaluate (1) 0.72 + 9, (2) 0.0625 + 5, (3) 0.000121 + 11
Solution:
1) 0.72 + 9 =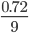 =0.08
=0.08
(Divided 72 by 9, get 8 as result now count decimal places in 0.72 that is ‘2’, hence place the decimal point on left of 08 that is, 0.08)
2) 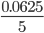 =0.0125
=0.0125
(Divided 625 by 5, get 125, place the decimal point on left of 0125 that is , 0.0125)
3) 0.000121 + 11=  = 0.000011
= 0.000011
(Divided 121 bt 11, get 11 as result, place decimal on left of 1.00011 that is, 0.000011.)
Division of a decimal fraction by a decimal fraction.
Example 6: Evaluate (1) 0.26 + 0.06 (2) 0.0077 + 0.11
Solution:
1) 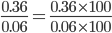
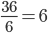
2) 0.0077 + 0.11 = 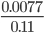
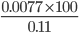
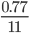 = 0.07
= 0.07
Decimal Fractions - Questions from Previous Year Papers
Decimal Fractions Aptitude
Decimal Fractions - Questions from Previous Year Papers





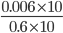

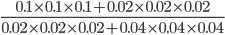
![\frac{(0.1)^{3}+(0.02)^{3}}{2^{3}[(0.1)^{3}+(0.02)^{3}]}=\frac{1}{8}](https://questionpaper.org/wp-content/plugins/latex/cache/tex_a37ab4c0fc28df690f5ef24bdad04285.gif)




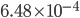
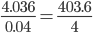
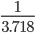
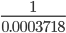
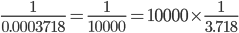
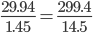
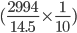
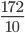
0 Comments